Leetcode: Unique Paths

Unique Paths

My Submissions

Total Accepted: 69248 Total Submissions: 201247 Difficulty: Medium

A robot is located at the top-left corner of a m x n grid (marked 'Start' in the diagram below).

The robot can only move either down or right at any point in time. The robot is trying to reach the bottom-right corner of the grid (marked 'Finish' in the diagram below).

How many possible unique paths are there?

Above is a 3 x 7 grid. How many possible unique paths are there?

Note: m and n will be at most 100.

Subscribe to see which companies asked this question

Hide Tags

 Array Dynamic Programming

Show Similar Problems

```````````````````````````````````````````````````````````````````````````````````````````````````````````````````````````````

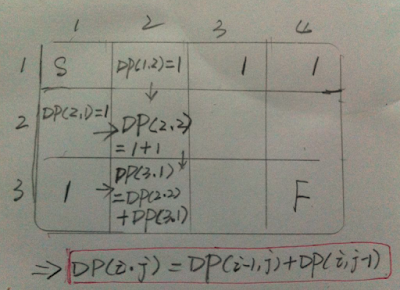
DP problem:

Find out the updating rules for the DP:

The updating rule is shown above. 

Note: the value for all boundary is 1. 

/////////////////////////////////////////////////////////////////////////

//////codes

class Solution {

public:

    int uniquePaths(int m, int n) {

        vector<vector<int>> dp;

        dp.resize(m);

        //set val for all boundary as 1

        for (int i=0;i<m;i++){

            dp[i].push_back(1);

        }

        for(int i=0;i<n;i++){

            dp[0].push_back(1);

        }

        for (int i=1;i<m;i++){

            for (int j=1;j<n;j++){

                int val=dp[i-1][j]+dp[i][j-1];

                dp[i].push_back(val);

            }

        }

        return dp[m-1][n-1];

        

    }

};